Two-loop evolution equations for light-ray operators

Contents lists available atScienceDirect

Physics Letters B

www.elsevier.com/locate/physletb

Two-loop evolution equations for light-ray operators

V.M. Braun

^a,∗

, A.N. Manashov

^a,b

aInstitutfürTheoretischePhysik,UniversitätRegensburg,D-93040Regensburg,Germany bDepartmentofTheoreticalPhysics,St.-PetersburgUniversity,199034,St.-Petersburg,Russia

a r t i c l e i n f o a b s t ra c t

Articlehistory:

Received4April2014 Accepted7May2014 Availableonline20May2014 Editor: A.Ringwald

QCDinnon-integerd=⁴−²space–timedimensionspossessesanontrivial criticalpointand enjoys exactscaleandconformalinvariance.Thissymmetryimposesnontrivialrestrictionsontheformofthe renormalizationgroupequationsforcompositeoperatorsinphysical(integer)dimensionsandallowsto reconstructfullkernelsfromtheireigenvalues(anomalousdimensions).Weusethistechniquetoderive two-loop evolutionequationsforflavor-nonsingletquark–antiquark light-rayoperatorsthatencode the scale dependenceofgeneralized hadronpartondistributions andlight-conedistributionamplitudesin themostcompactform.

©^{2014TheAuthors.PublishedbyElsevierB.V.ThisisanopenaccessarticleundertheCCBYlicense} (http://creativecommons.org/licenses/by/3.0/).FundedbySCOAP³.

1. Studies of hard exclusive reactions contribute significantly tothe research program atall major existing andplanned accel- erator facilities. The relevant nonperturbative input in such pro- cessesinvolvesoperatormatrixelementsbetweenstateswithdif- ferentmomenta, dubbedgeneralized partondistributions (GPDs), orvacuum-to-hadronmatrixelementsrelatedtolight-fronthadron wave functions at small transverse separations, the distribution amplitudes(DAs).Scale dependenceofthesedistributions isgov- ernedbythe renormalizationgroup (RG) equationsforthecorre- sponding(nonlocal)operatorsandhastobe calculatedtoasuffi- cientlyhighorderinperturbationtheoryinordertomaketheQCD descriptionofexclusivereactionsfullyquantitative.Atpresent,the evolution equations for GPDs (and DAs) are known to the two- loopaccuracy[1–3],oneorderlesscomparedtothecorresponding

“inclusive”distributionsthatinvolveforwardmatrixelements[4,5]

andclosingthisgapisdesirable.Thedirectcalculationisverychal- lenging,andalsofinding a suitable representationfor theresults maybecome a problem asthe two-loop evolution equations for GPDsarealreadyverycumbersome.

Ithas beenknown forsome time [6]that conformal symme- try of the QCD Lagrangian allows one to restore full evolution kernels atgiven orderof perturbation theory fromthe spectrum ofanomalous dimensionsat the sameorder, and the calculation of the special conformal anomaly at one order less. This result was used to calculate the complete two-loop mixing matrix for twist-twooperatorsinQCD[7–9],andderivethetwo-loopevolu- tionkernels inmomentumspaceforthe GPDs[1–3].InRef. [10]

we havesuggested an alternative technique, thedifference being

*

Correspondingauthor.

thatinsteadofstudyingconformalsymmetrybreakinginthephys- ical theory [7–9] we make use ofthe exactconformal symmetry of a modified theory – QCD ind=⁴−²

dimensionsat critical coupling. Exact conformal symmetry allows one to use algebraic group-theory methodsto resolve the constraints on the operator mixingandalsosuggeststheoptimalrepresentationfortheresults intermsoflight-rayoperators.Inthiswayadelicateprocedureof the restorationofthe evolutionkernels fromtheresults forlocal operatorsiscompletelyavoided.Weexpectthatthesefeatureswill becomeincreasinglyadvantageousinhigherorders.

This modified approach was illustrated in [10] on several ex- amples to the two- and three-loop accuracy for scalar theories.

Application to gauge theories, inparticular QCD, involves several subtleties that are considered in thiswork. The main newresult are thetwo-loop evolutionequationsforflavor-nonsingletquark–

antiquarklight-rayoperators thatencodethescaledependenceof generalizedhadronpartondistributionsandlight-conedistribution amplitudesinthemostcompactform.

2. Beforegoingovertotechnicaldetails,letusfirstdescribethe generalstructureoftheapproachandtheresultsonamorequal- itativelevel.Inordertomakeuseofthe(approximate)conformal symmetry of QCD it is natural to use a coordinate-space repre- sentation in which the symmetry transformations have a simple form[11].Therelevantobjectsarelight-rayoperatorsthatcanbe understood asgeneratingfunctionsforthe renormalizedleading- twistlocaloperators:

[

O

](

^x

;

^z1

,

z₂

) ≡

¯

q

(

x

+

^z1n

)

nq/

(

x

+

^z2n

)

≡

m,k

z^m₁z^k₂ m

!

^k

!

D^m_n^q

¯ (

x

)

n/

D^k_nq

(

x

)

.

(1)

http://dx.doi.org/10.1016/j.physletb.2014.05.037

0370-2693/©^{2014TheAuthors.PublishedbyElsevierB.V.ThisisanopenaccessarticleundertheCCBYlicense}(http://creativecommons.org/licenses/by/3.0/).Fundedby SCOAP³.

Hereq(x)isaquarkfield,theWilsonlineisimplied betweenthe quark fields onthe light-cone, Dn=ⁿμDμ is a covariantderiva- tive,nμisanauxiliary light-likevector,n²=^{0,thatensures sym-} metrizationandsubtractionoftracesoflocaloperators.Thesquare brackets[. . .]^standfortherenormalizationusingdimensionalreg- ularization and MS subtraction. We will tacitly assume that the quarkandantiquarkhavedifferentflavorsothatthereisnomix- ingwithgluonoperators.Inmostsituationstheoverallcoordinate xμ isirrelevant andcanbe putto zero;we willoftenabbreviate O(^z1,z₂)≡O(⁰;^z1,z₂).

Light-rayoperatorssatisfyarenormalization-groupequation of theform[12]

M

∂

M

+ β(

g

)∂

g

+ H

O

(

z₁

,

z₂

)

=

⁰

,

(2)

where H ^{is an integral operator actingon the light-cone coordi-} natesofthefields.Itcanbewrittenas

H[

O

](

^z1

,

z₂

) =

1

0

d

α

1

0

d

β

h

( α , β)[

O

]

z^α₁₂

,

z^β₂₁

,

(3)

where

z^α₁₂

≡

^z1

α ¯ +

^z2

α , α ¯ =

¹

− α ,

(4) andh(

α

,β)isacertainweightfunction(kernel).

One can show, see e.g. [10], that the powers [O](^z1,z₂)→ (z1−^z2)^N areeigenfunctionsoftheevolutionoperatorH^,andthe correspondingeigenvalues

γ

N

=

d

α

d

β

h

( α , β)(

1

− α − β)

^N−1 (5) arenothingelseastheanomalousdimensionsoflocaloperatorsof spinN (withN−1 derivatives).

In generalthe function h(

α

,β) is a function of two variables andthereforethe knowledgeof theanomalous dimensions

γ

N is not sufficient to fix it. However, if the theory is conformally in- variantthentotheone loopaccuracy H^{mustcommute withthe} generatorsoftheSL(2)transformations[H,^S(α⁰⁾]=^0,where

S⁽₊⁰⁾

=

^z1²

∂

z₁

+

^z22

∂

z₂

+

²

(

z1

+

^z2

),

S⁽₀⁰⁾

=

z1

∂

z₁

+

z2

∂

z₂

+

2

,

S⁽₋⁰⁾

= −∂

z₁

− ∂

z₂

.

(6) Inthiscaseitcanbeshownthatthefunctionh(

α

,β)(uptotrivial terms∼δ(

α

)δ(β)that correspond to theunit operator)takesthe form[13]

h

( α , β) = ¯

^h

( τ ), τ ₌ α β

¯

α β ¯

⁽⁷⁾

andiseffectivelyafunctionofonevariable

τ

calledtheconformal ratio.Thisfunctioncaneasily bereconstructed fromitsmoments (5),aliasfromtheanomalousdimensions.

ConformalsymmetryofQCDisbrokenbyquantumcorrections whichimpliesthatthesymmetryoftheevolutionequationsislost atthetwo-looplevel.Inotherwords,writingtheevolutionkernel asanexpansioninthecouplingconstant

H =

^as

H

⁽¹⁾

+

^a2_s

H

⁽²⁾

+ . . .

→

^h

( α , β) =

^ash⁽¹⁾

( α , β) +

^a2_s^h(2)

( α , β) + . . . ,

(8) where as=

α

s/(4

π

), we expect that h⁽¹⁾(

α

,β) only dependson theconformalratiowhereas higher-ordercontributionsremainto benontrivialfunctionsoftwovariables

α

andβ.

Thispredictionisconfirmedbytheexplicitcalculation[12]:

H

^(1)f

(

z₁

,

z₂

)

=

^4CF

1 0

d

α α ^¯ α

2f

(

z₁

,

z₂

) −

^f

z^α₁₂

,

z₂

−

^f

z₁

,

z^β₂₁

−

1

0

d

α

¯

α

0

d

β

f

z^α₁₂

,

z^β₂₁

+

¹

2f

(

z₁

,

z₂

) .

(9) Thecorrespondingone-loopkernelh⁽¹⁾(

α

,β)canbewritteninthe following,remarkablysimpleform[13]

h⁽¹⁾

( α , β) = −

^4CF

δ

₊

( τ ) + θ (

1

− τ ) −

¹

2

δ( α )δ(β)

,

(10)

wheretheregularizedδ-function,δ₊(

τ

),isdefinedas

d

α

d

β δ

₊

( τ )

f

z^α₁₂

,

z^β₂₁

≡

1

0

d

α

1

0

d

β δ( τ )

f

z^α₁₂

,

z^β₂₁

−

^f

(

z₁

,

z₂

)

= −

1

0

d

α α ^¯ α

2f

(

z₁

,

z₂

) −

^f

z^α₁₂

,

z₂

−

^f

z₁

,

z^α₂₁

.

(11)

TakingappropriatematrixelementsandmakingaFouriertransfor- mation tothe momentum fraction spaceone can check that the expression inEq. (10) reproduces all classical leading-order (LO) QCDevolutionequations:DGLAPequationforpartondistributions, ERBLequationforthemesonlight-coneDAs,andthegeneralevo- lutionequationforGPDs.

The two-loop kernel h⁽²⁾(

α

,β) contains contributions of two colorstructuresandatermproportionaltotheQCDbetafunction, h⁽²⁾

( α , β) =

8C²_Fh⁽₁²⁾

( α , β) +

4CFCAh⁽₂²⁾

( α , β)

+

^4b0C_Fh⁽₃²⁾

( α , β).

(12)

Let usexplain how it can be calculated.The idea ofRef. [10]

is to consider a modified theory, QCD in non-integer d=⁴−²

dimensions.Inthistheorytheβ-functionhastheform

β(

a

) =

^M

∂

Ma

=

^2a

− −

^b0a

+

O

a²

,

b0

=

¹¹

3 Nc

−

²

3n_f

,

(13)

andforalargenumberofflavorsn_f thereexistsacriticalcoupling a^∗_s=

α

_s^∗/(4

π

)∼

suchthat β(a^∗_s)=^{0.Thetheorythusenjoysex-} actscaleinvariance[14,15]andonecanargue(seebelow)thatfull conformalinvarianceisalsopresent.¹Asaconsequence,therenor- malizationgroup equationsare exactlyconformally invariant: the evolution kernels commute withthe generators ofthe conformal group. Thegeneratorsare, however,modifiedby quantumcorrec- tionsascomparedtotheircanonicalexpressions(6):

S_α

=

^S(α⁰⁾

+

^a∗s

S_α⁽¹⁾

+

a^∗_s

2

S⁽_α²⁾

+ . . .

(14)

1 Formallythegauge-fixed QCDLagrangiancontainstwocharges,thecoupling andthegaugeparameter.Thecorrespondingβ-function,βξ=M∂Mξ,vanishesin theLandaugauge,ξ=0,sothatallGreenfunctionsarescale-invariantatthecrit- icalpointinthisgauge;βξ alsodropsoutoftheRGequationsforthecorrelation functionsofgauge-invariantoperators.

Onecanshowthat

S₋

=

^S(₋⁰⁾

,

S0

=

^S(₀⁰⁾

− ₊

¹

2

H

a^∗_s

, H

a^∗_s

=

^a∗s

H

⁽¹⁾

+ . . .

S₊

=

^S(₊⁰⁾

+ (

z1

+

^z2

)

− ₊

¹

2a^∗_s

H

⁽¹⁾

+

^a∗s

(

z1

−

^z2

)Δ

₊

+

O

²

,

(15)

where

Δ

₊

[

O

] (

z1

,

z2

)

= −

^2CF

1

0

d

α α ¯

α ⁺

^ln

α _[

O

]

z^α₁₂

,

z2

− [

O

]

z1

,

z^α₂₁

(16)

i.e.the generator S₋ is not modified, the deformation of S₀ can be calculated exactly in terms of the evolution operator (to all orders in perturbation theory) [10], whereas the deformation of S₊ isnontrivialandhastobecalculatedexplicitlyorderbyorder, totherequired accuracy.The one-loop expressionshownin (15), (16)isderived below,it isa newresult.From thepure technical pointofview,thiscalculationreplacesevaluationoftheconformal anomaly in the theory with brokensymmetry in integer dimen- sionsviatheconformalWardidentities(CWI)intheapproachdue toD. Müller[6].

Conformalsymmetry ofthe modified QCD atthe critical cou- plingimpliesthatthegenerators(15)satisfy theusualSL(2)com- mutationrelations

[

^S0

,

S_±

] = ±

^S±

, [

^S+

,

S₋

] =

^2S0

.

(17) Expandingtheminpowersofthecouplinga^∗_s oneobtainsanested setofcommutatorrelations[10]

S⁽₊⁰⁾

, H

⁽¹⁾

=

⁰

,

S⁽₊⁰⁾

,H

⁽²⁾

=

H

⁽¹⁾

, Δ

S⁽₊¹⁾

,

S⁽₊⁰⁾

,H

⁽³⁾

=

H

⁽¹⁾

, Δ

S⁽₊²⁾

+

H

⁽²⁾

, Δ

S⁽₊¹⁾

,

(18)

etc.NotethatthecommutatorofthecanonicalgeneratorS⁽₊⁰⁾with the evolution kernel at order k on the l.h.s. of each equation is givenin terms of theevolution kernels H^{(k) and the}corrections to the generators ΔS⁽₊^m) at one order less, m≤^k−^{1. The com-} mutationrelationsEq.(18)canbeviewedas,essentially,inhomo- geneousfirst-orderdifferentialequationsontheevolutionkernels.

TheirsolutiondeterminesH^(k)uptoanSL(2)-invariantterm(solu- tionofahomogeneousequation[H⁽_inv^k),S⁽α⁰⁾]=^{0),whichcan,again,} berestoredfromthespectrumoftheanomalousdimensions.This procedureisdescribedindetailforscalartheoriesinRef.[10].

Last but not least, in MS-like schemes the evolution kernels (anomalousdimensions)donotdependonthespace–timedimen- sionby construction. Indeed,therenormalization Z^{factors relat-} ing the renormalized and bare light-ray operators [O](^z1,z₂)= ZO(z1,z2)aregivenbytheexpansion

Z =

¹

+

^∞

j=1

^−j

∞ k=j

a^k_s

Z

jk

,

(19)

whereZjkhavetheintegral representationsimilarto(3)interms offunctionsoftwo variables, Zjk(

α

,β) thatdonot dependon

. Thus,eliminatingthe

-dependenceoftheexpressions derivedin thed-dimensional (conformal)theory forthe criticalcoupling by theexpansion = −^b0a^∗_s+O(^a∗s²)allows onetorestore theevo- lution kernels for the theory in integer dimensions for arbitrary couplinga^∗_s→^as;thisrewritingissimpleandexacttoallorders.

3. The statement ofconformal invarianceof QCDin ddimen- sionsatthecriticalcouplingisnottrivial.Itisbelievedthat“phys- icallyreasonable”scale-invarianttheoriesare alsoconformallyin- variant,seeRef.[16] fora discussion,however,tothebestofour knowledgethereisnoproofofthisstatementford>2 dimensions (buttherearenocounterexamplesaswell).Innon-gaugetheories conformalinvariancefortheGreenfunctionsofbasicfieldscanbe checkedinperturbativeexpansions[17].Ingaugetheoriesconfor- malinvariancedoesnotholdforthecorrelatorsofbasicfieldsand can be expected only forthe Green functionsof gauge-invariant operators. Forlocal compositeoperators a proof ofconformal in- variance is based on the analysis of pair counterterms for the product of the trace of energy-momentum tensor and local op- erators [18]. This analysis is beyond the scope of this Letter; it becomes rather complicated in gauge theories due to mixing of gauge invariant operators with BRST variations and equation of motion(EOM)operators[19].

Ashort commentmay,nevertheless, berelevant. LetON bea gauge-invariantmultiplicativelyrenormalizableoperator

M

∂

M

+ β(

a

)∂

a

+ γ

N

(

a

)

[

ON

] =

⁰

,

(20) where

γ

N(a) is the anomalous dimension. As a consequence, it possessesacertain(critical)dimensionforthefine-tunedvalueof thecoupling(criticalpoint)a=^a∗,β(a_∗)=^0:

i

D

, [

ON

] (

x

)

=

x

∂

_x

+ Δ

^∗_N

[

ON

] (

x

),

(21) where D is the operator of dilatations, ΔN is the canonical di- mension of the operator ON, and Δ^∗_N =ΔN+

γ

_N^∗ is the scaling dimension,

γ

_N^∗=

γ

N(a_∗).

The statement that [ON](^x) becomes a conformal operator at thecriticalpoint,aswidelyexpected,meansthatactionofthegen- eratorofspecialconformaltransformationsonthisoperatortakes theform

i

K^μ

, [

ON

] (

x

)

=

2x^μ

(

x

∂) −

^x2

∂

^μ

+

²

Δ

^∗_Nx^μ

+

^2xν

n^μ

∂

∂

n^ν

−

ⁿν

∂

∂

n_μ

[

ON

] (

x

).

(22) Equivalently,acorrelationfunctionofsuchoperatorsatthecritical pointmustsatisfytheWardidentity

K_x^μ₁

+ . . . +

^Kx^μn

[

O1

](

^x1

) . . .[

On

](

^xn

)

=

⁰

,

(23)

whereitisassumedthatallspace–timepointsxiaredifferent.Cal- culatingthel.h.s.inperturbationtheory(seeRef.[18])andmaking useofthedilatationWardidentityproducestheexpressionofthe form

K_x^μ₁

+ . . . +

^Kx^μn

[

O1

] (

x1

) . . . [

On

] (

xn

)

=

N

i=1

[

O1

] (

x1

) . . .

_O

^μ_i

(

xi

) . . . [

On

] (

xn

)

,

(24)

whereOi(xi)arelocaloperatorinsertionsthatinvolveseveralcon- tributions:EOMoperators,operatorsrepresentingaBRSTvariation of another operator, andgauge-invariant operators. The first two canbeneglectedastheydonotcontributetothecorrelationfunc- tion(assumingxj =^xk,forall j =^{k).The lastonescanfurtherbe} expanded in terms of gauge invariant operators [O^μ_iq](^xi) witha certaincriticaldimension,

O

^μ_i

(

x_i

) =

q

cq

(

a

)

O^μ_iq

(

x_i

).

Dilatationinvarianceimpliesthat thebothsidesofEq.(24)must have the same scaling dimension at the critical point. Note that applicationof Kμ lowersthescaling dimensionofan operatorby one. As a consequence,foreach contributionon the r.h.s., either c_q(a_∗)vanishes, orthescalingdimensionsof[O^μ_iq]^and[Oi]^must differby one, dimO^μ_iq=^dimOi−^{1, toall orders of}perturbation theory. If such operators do not exist, then all coefficient cq(a) havetovanishatthecritical pointthat impliesconformal invari- ance Eq.(23). Forthe leading-twist operators that are subject of thisLetter,absenceofoperatorswiththesameanomalousdimen- sionandthecanonicaldimensionlessbyoneiseasytoverify.For thegeneralcase,it wouldbequiteunexpected thattwo different operatorshavethesameanomalousdimensionifthey arenotre- lated by some exact symmetry, although existence of such pairs cannotbeexcluded.

4. The correction ΔS₊, S₊=^S₊⁽⁰⁾+ΔS₊, to the generator of special conformal transformations atthe criticalpoint inQCD in ddimensions(inthelight-rayoperatorrepresentation)canbede- rivedfromtheanalysisofCWIsforsuitablecorrelation functions.

ThestandardprocedureistoconsidertheGreenfunctionsoftwist- twooperators withquark fields,seeRefs. [3,7](ford=^{4), which} arenotgaugeinvariantthatcomplicatestheanalysis.Thisdifficulty canbeavoidedbyconsidering,instead,thecorrelatoroftwolight- rayoperatorsthatdependondifferentauxiliaryvectorsnand^n:¯ G

(

x

,

z

,

w

) =

O⁽ⁿ⁾

(

0

,

z

)

O^(¯n)

(

x

,

w

)

=

N⁻¹

D

Φ

e^−SR(Φ)

O⁽ⁿ⁾

(

0

,

z

)

O^(¯n)

(

x

,

w

),

(25) where Φ ≡ {^A,q,^q¯,c,^c¯} ^{is the set of} fundamental fields, z≡ {^z1,z2}^{, w}≡ {^w1,w2}^{andweassumethattheauxiliarylight-like} vectorsarenormalizedas(n· ¯ⁿ)=^1.

TheQCDLagrangianind=⁴−²

dimensionalEuclideanspace–

timeincovariantgaugehastheform² L

= ¯

q

(

/

∂ −

ig

/

A

)

q

+

¹

4F_μν^a F^a,μν

+ ∂

_μc

¯

^a

D^μc

a

+

¹ 2

ξ

∂

A^a

2

.

(26) Therenormalizedaction SR isobtainedfrom(26)by thereplace- ment

Φ → Φ

0

=

^ZΦ

Φ,

g

→

^g0

=

^MZgg

,

ξ → ξ

0

=

^Zξ

ξ,

(27)

i.e. S_R(Φ,g,ξ )=^S(Φ0,g₀,ξ0). Note that we do not send

→⁰ in the renormalized correlation functions so that they explicitly dependon .

TheformoftheCWIissimplerforthespecialchoice (n·^x)= (¯n·^x)=^{0 thatweacceptforthis}calculation.Forthelocalconfor- maloperatorsdefinedwithrespecttotheⁿ¯ ^vector,O^(¯_Nⁿ⁾(x),cf.[11], itfollowsfrom Eq.(22)that

i

(¯

^nK

),

O^(¯_Nⁿ⁾

(

x

)

= −

^x2

(

ⁿ

¯ ∂

x

)

O^(¯_Nⁿ⁾

(

x

).

(28) Goingovertothelight-rayoperatorsoneobtains,therefore i

(¯

^nK

),

O^(¯n)

(

x

,

w

)

= −

^x2

(

ⁿ

¯ ∂

x

)

O^(¯n)

(

x

,

w

).

(29) Thus conformal invarianceof thecorrelation function (25)atthe criticalpointimpliestheconstraint

2 OurnotationfollowscloselyRef.[20]

i 2

(

^nK

¯ ),

O⁽ⁿ⁾

(

0

,

z

)

O^(¯n)

(

x

,

w

) +

O⁽ⁿ⁾

(

0

,

z

)

(¯

^nK

),

O^(¯n)

(

x

,

w

)

=

S⁽₊^z)

−

¹ 2x²

(

n

¯ ∂

x

)

G

(

x

,

z

,

w

) =

0

,

(30) wherethesuperscriptS⁽₊^z)remindsthatitisadifferentialoperator actingon z₁,z₂ coordinates.

ExplicitexpressionforS⁽₊^z)canbederivedfromtheCWI 0

= −

δ

₊S_R

O⁽ⁿ⁾

(

z

)

O^(¯n)

(

x

,

w

) +

δ

₊

O⁽ⁿ⁾

(

z

)

O^(¯n)

(

x

,

w

) +

O⁽ⁿ⁾

(

z

) δ

₊

O^(¯n)

(

x

,

w

)

,

(31)

where[O](^z1,z2)≡ [O](^x=⁰;^z1,z2),thatfollowsfrominvariance ofthecorrelation function(25)undera changeofvariablesΦ→ Φ+δ₊Φ inthepathintegral,

δ

₊q

(

x

) = ¯

^nμ

2x_μ

(

x

∂) −

^x2

∂

_μ

+

²

Δ

qx_μ

q

(

x

) +

¹

2

[ γ

_μ

,

/x

]

^q

(

x

)

, δ

₊A_ρ

(

x

) = ¯

^nμ

2x_μ

(

x

∂) −

^x2

∂

μ

+

²

Δ

Ax_μ

A_ρ

(

x

) +

^2gμρ

(

x A

) −

^2xρA_μ

(

x

)

, δ

₊c

(

x

) = ¯

^nμ

2x_μ

(

x

∂) −

^x2

∂

μ

+

²

Δ

cx_μ

c

(

x

), δ

₊c

¯ (

x

) = ¯

^nμ

2x_μ

(

x

∂) −

^x2

∂

μ

+

²

Δ

c_¯x_μ

¯

c

(

x

).

(32)

ThechoiceoftheparametersΔΦ isamatterofconvenience.They can betaken, e.g.,equaltothecanonicaldimensionsofthefields indspace–timedimensions,asin[10].ForQCDadifferentchoice proves tobemoreconvenient:Δq=³/2−

,ΔA=^1,Δc_¯=^{2 and} Δc=^{0.Inthiscasethe quark partoftheLagrangian isinvariant} andvariationoftheactiontakestheform

δ

₊SR

=

4

d^dx

(

xn

¯ )(

LA

+

Lξ

+

Lghost

) +

²

(

d

−

²

)¯

n^μ

d^dx

Z²_c

¯

c D_μc

−

¹

ξ

^Aμ

(∂

A

)

.

(33)

Thereasonforchoosingdifferentscalingdimensionsfortheghost andanti-ghostfieldsisthatinthiscasethelastterm∼(d−2)that doesnotvanishinfourdimensionsisaBRSTvariation[1]

Z²_c

¯

^caDμc^a

−

¹

ξ

^A

aμ

∂

A^a

= δ

BRST

^c

¯

^aAa_μ

.

(34)

Hence,itdoesnotcontributetothevariationof(25).

In this work we are interested in the one-loop correction to thegeneratorS₊.Tothisaccuracy,obviously,theghostLagrangian Lghost andgluonself-interactiondonot contributei.e.wehaveto keep termsquadraticingluon fieldsonly. Oneobtainsaftersome algebra

δ

₊SR

= −

²

d^dx

(

xn

¯ )

A^a_αK^αβA^a_β

+

1

+

¹

ξ

n A

¯

^a

∂

A^a

+ . . . ,

(35)

wherethe ellipsesstands forthe termsthatare irrelevant atone looporderand

K^αβ

=

^gαβ

∂

²

− ∂

^α

∂

^β

1

−

¹

ξ

(36)

is nothingbutthe inversegluon propagator(with a minus sign).

Moreover, asfollows fromEq.(34), thelast term ∼(^{n A}¯ )(∂A) can be written asa combinationoftheBRST variation andthe ghost term, so that it doesnot contribute to the one-loop accuracy as well. Thus, to our accuracy, the insertion of δ₊SR generates an effectivevertexinsertion−²

(¯nx)A^aα(x)KαβA^a_β(x)inagluonline inoneloopdiagrams:

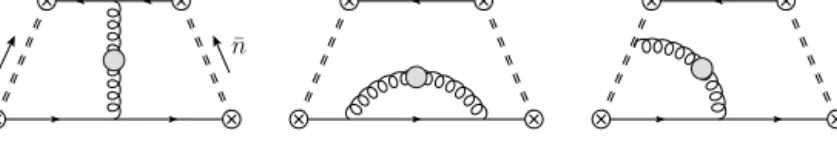
Fig. 1.One-loopdiagramscontributingtothecorrelationfunctionoftwolight-rayoperatorswithaninsertionofδ+SR (greyblobs).Wilsonlinesbetweenthequarkfields areshownbythedasheddoublelines.

=

²

n

¯ · (

x

+

^y

)

(37)

Feynman diagrams contributing to the correlation function δ+S_R[O⁽ⁿ⁾](^z)[O^(¯n)](^x,w)^{onther.h.s.oftheCWI(31)areshown} inFig. 1.Sincetheδ₊SR insertion bringsthefactor

g²,weonly needthedivergentparts.Thecalculationisveryeasy.Fordefinite- ness let us choose the Feynman gauge, ξ=^{1, and consider the} first diagram in Fig. 1 as an example. This diagram, potentially, hastwodivergent subgraphs—leftandright—buttherightone doesnot contribute dueto our choice (¯n,x)=^{0, takinginto ac-} countthattheoperatorO^(¯n)(x,w)onlyinvolvesquarkfieldsalong thelineⁿ¯^{μ.Thanksto(37)thedivergentpartoftheleftsubgraph} coincides upto a prefactor2

(ⁿ¯,y+^y),withthe corresponding counterterm for the operator O⁽ⁿ⁾(z1,z2), which is known from Ref.[12].Oneobtainsforthiscontribution

(

nn

¯ ) α

s

π

^CFH

(+)

(

z1

+

^z2

)

G⁽⁰⁾

(

x

,

z

,

w

),

(38)

whereG⁽⁰⁾(x,z,w) isthe tree-levelcorrelation function(25) and theintegraloperatorH^{(+)isdefinedasfollows:}

H^(+)f

(

z₁

,

z₂

) =

1

0

d

α

¯

α

0

d

β

f

z^α₁₂

,

z^β₂₁

.

(39)

TheotherdiagramsinFig. 1,similarly,arewrittenintermsofcon- tributionsofthecorrespondingcountertermstoO⁽ⁿ⁾(z1,z2)deco- ratedbymultiplicativefactors.Forthesumofalltermsoneobtains

δ

₊S_R

O⁽ⁿ⁾

(

0

,

z

)

O^(¯n)

(

x

,

w

)

= (

nⁿ

¯ ) α

s

π

^CF

−{

_H

₁

,

z₁

} − {

_H

₂

,

z₂

}

+

H⁽⁺⁾

−

¹ 2

(

z1

+

^z2

) +

^z12_H

G⁽⁰⁾

(

x

,

z

,

w

)

+

O

( ),

(40)

where{∗,∗}^standsforananticommutatorand

H

1f

(

z1

,

z2

) =

1

0

d

α α ^¯ α

f

(

z1

,

z2

) −

f

z^α₁₂

,

z2

,

H

2f

(

z₁

,

z₂

) =

1

0

d

α α ^¯ α

f

(

z₁

,

z₂

) −

^f

z₁

,

z^α₂₁

,

H

f

(

z1

,

z2

) =

1

0

d

α

ln

α

f

z^α₁₂

,

z2

−

f

z1

,

z^α₂₁

.

(41)

Theone-loopevolutionoperatorH^{(1)inEq.(8)iswritteninterms} ofthesekernelsas

H

⁽¹⁾

=

^4CF

_H

₁

+

_H

₂

−

H⁽⁺⁾

+

¹ 2

.

(42)

ThisrepresentationisequivalenttotheoneinEq.(10)and,asitis easytoshow,H^{(1)commuteswith S(}₊^0).

Next, we have to consider the second contribution on the r.h.s. of the CWI (31), which involves the conformal variation of [O^(¯n)](^x,w),

δ

₊

O^(¯n)

(

x

,

w

) = Zδ

+O^(¯n)

(

x

,

w

)

= Z

−

^x2

(¯

n

∂

_x

)

O^(¯n)

(

x

,

w

)

= −

^x2

(¯

n

∂

_x

)

O^(¯n)

(

x

,

w

),

(43)

and,finally,thethirdcontribution

δ

₊

O⁽ⁿ⁾

(

z

) =

²

(

nn

¯ ) Z δ

₊

O⁽ⁿ⁾

(

z

)

=

²

(

nn

¯ ) Z

S⁽₊⁰⁾

− (

z₁

+

^z2

) Z

⁻¹

O⁽ⁿ⁾

(

z

)

=

2

(

nn

¯ )

S⁽₊⁰⁾

− (

z1

+

z2

)

−

^as 2

H

⁽¹⁾

,

z1

+

^z2

O⁽ⁿ⁾

(

z

).

(44)

ThislastcontributionisdiscussedindetailinRef.[10] wherethe chain of equations in (44) is explained. Collecting all terms we obtain the resultquoted in Eq.(15). Notethat this expression is differentfromthecorrespondingresultinscalarfieldtheories, S₊

=

^S(₊⁰⁾

+ (

z₁

+

^z2

)

− +

¹ 2a_∗

H

⁽¹⁾

,

seeRef.[10].

5. WeproceedtocalculatetheNLOevolutionkernels(12)mak- ing use of the commutator relation [^S(₊⁰⁾,H⁽²⁾]= [H⁽¹⁾,S⁽₊¹⁾]^, Eq.(18).NotethatS⁽₊¹⁾(15)containstermsinb0andCF.³Hence thecommutator [^S(₊¹⁾,H⁽¹⁾] ^{containstwo color}structures, b₀C_F andC²_F,respectively.ItfollowsthatthekernelCFCAh⁽₂²⁾(

α

,β)(12) satisfies the homogeneous equation [^S(₊⁰⁾,H⁽₂²⁾]= ^{0, alias it is} SL(2)-invariantandcanbewrittenasafunctionoftheconformal ratio,h⁽₂²⁾(

α

,β)=^h(₂²⁾(

τ

).

Calculatingthecommutatorweobtainaftersomealgebra

S⁽₊¹⁾

, H

⁽¹⁾

=

^8C2F

A

1

+

^4CFb0

A

3

,

(45) whereA1 andA3 areintegraloperatorsoftheform

A

if

(

z₁

,

z₂

) =

^z12

1

0

d

α

A_i

( α )

f

z^α₁₂

,

z₂

−

^f

z₁

,

z^α₂₁

+

z12

1

0

d

α

¯

α

0

d

β

Bi

( α , β)

f

z^α₁₂

,

z^β₂₁

,

with

3 Totheone-loopaccuracyonecanreplace=(4−^d/2)by−^b0a^∗_s.