Introduction to surgery theory (Lecture II)

(1)

Introduction to surgery theory (Lecture II)

Wolfgang Lück Bonn Germany

email wolfgang.lueck@him.uni-bonn.de http://131.220.77.52/lueck/

Oberwolfach, October 2017

Wolfgang Lück (Bonn) Introduction to surgery theory Oberwolfach, October 2017 1 / 38

(2)

Outline

State theexistence problemanduniqueness problemin surgery theory.

Explain the notion ofPoincaré complexand ofSpivak normal fibration.

Introduce thesurgery problem, thesurgery stepandthe surgery obstruction.

Explain thesurgery exact sequenceand its applications to topological rigidity.

(3)

The goal of surgery theory

Problem (Existence)

Let X be a space. When is X homotopy equivalent to a closed manifold?

Problem (Uniqueness)

Let M and N be two closed manifolds. Are they isomorphic?

(4)

For simplicity we will mostly work withorientable connected closed manifolds.

We can work withtopologicalmanifolds,PL-manifolds orsmooth manifolds and then isomorphic meanshomeomorphic,

PL-homeomorphicordiffeomorphic.

We will begin with the existence problem. We will later see that the uniqueness problem can be interpreted as a relative existence problem thanks to the s-Cobordism Theorem.

(5)

Poincaré complexes

A closed manifold carries the structure of a finiteCW-complex.

Hence we assume in the sequel in the existence problem thatX itself is already aCW-complex.

Fix a natural numbern≥4. Then every finitely presented group occurs as fundamental group of a closedn-dimensional manifold.

Since the fundamental group of a finiteCW-complex is finitely presented, we get no constraints on the fundamental group.

Exercise (Fundamental groups of closed 3-manifolds)

Let G be the fundamental group of a closed3-manifold. Show that thendim_Q(H₂(G;Q))≤dim_Q(H₁(G;Q))holds.

(6)

LetM be a (connected orientable) closedn-dimensional manifold.

ThenH_n(M;Z)is infinite cyclic. If[M]∈H_n(M;Z)is a generator, then the cap product with[M]yields fork ∈Zisomorphisms

− ∩[M] : H^n−k(M;Z)−→^∼⁼ H_k(M;Z).

ObviouslyX has to satisfy the same property if it is homotopy equivalent toM.

There is a much more sophisticated Poincaré duality behind the result above which we will explain next.

Recall that a (not necessarily commutative)ring with involutionR is ringRwith aninvolution of rings

:R→R, r 7→r,

i.e., a map satisfyingr =r,r +s =r +s,r ·s=s·r and 1=1 for r,s∈R.

(7)

Our main example is the involution on the group ringZGfor a groupGdefined by sendingP

g∈Ga_g·gtoP

g∈Ga_g·g⁻¹. LetM be a leftR-module. ThenM^∗ :=hom_R(M,R)carries a canonical rightR-module structure given by(fr)(m) =f(m)·r for a homomorphism of leftR-modulesf:M →Randm∈M. The involution allows us to viewM^∗=hom_R(M;R)as a leftR-module, namely, definerf forr ∈Randf ∈M^∗ by(rf)(m) :=f(m)·r for m∈M.

Given anR-chain complex of leftR-modulesC∗ andn∈Z, we define itsdual chain complexC^n−∗to be the chain complex of left R-modules whosep-th chain module is hom_R(Cn−p,R)and whosep-th differential is given by

(−1)^n−p+1·hom_R(cn−p+1,id) : (C^n−∗)p =hom_R(Cn−p,R)

→(C^n−∗)p−1=hom_R(C_n−p+1,R).

(8)

Definition (Finite Poincaré complex)

A (connected) finiten-dimensionalCW-complexX is afinite

n-dimensional Poincaré complexif there is[X]∈H_n(X;Z)such that the inducedZπ-chain map

− ∩[X] : C^n−∗(X)e →C∗(X)e is aZπ-chain homotopy equivalence.

If we apply id_Z⊗Zπ−, we obtain aZ-chain homotopy equivalence C^n−∗(X)→C∗(X)

which induces after taking homology the Poincaré duality isomorphism− ∩[X] : H^n−k(M;Z)−→^∼⁼ H_k(M;Z)from above.

(9)

Theorem (Closed manifolds are Poincaré complexes)

A closed n-dimensional manifold M is a finite n-dimensional Poincaré complex.

We conclude that a finiten-dimensionalCW-complexX is homotopy equivalent to a closedn-dimensional manifold only if it is up to homotopy a finiten-dimensional Poincaré complex.

Exercise (Poincaré chain homotopy equivalence forS¹)

Equip S¹with the CW -structure with two cells. Write down explicitly a Z[π₁(S¹)]-chain homotopy equivalence

C^1−∗(Sf¹)→C_∗(Sf¹)

after appropriate identifications of the chain modules withZ[π1(S¹)].

(10)

The Spivak normal fibration

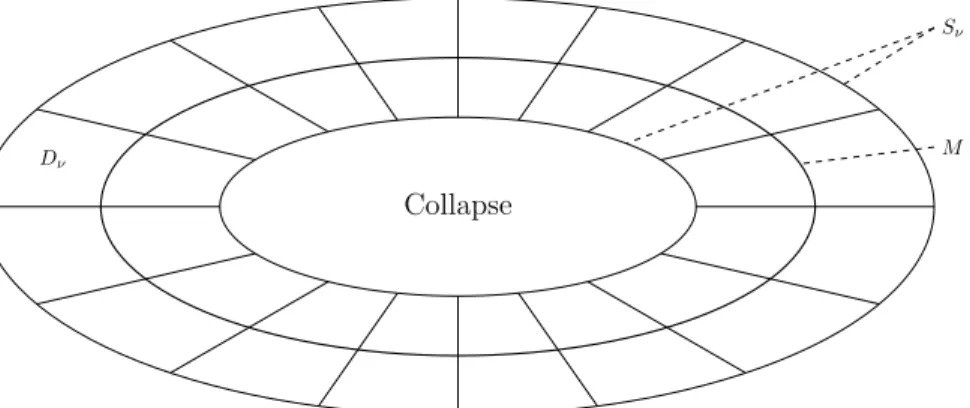
We briefly recall thePontryagin-Thom constructionfor a closed n-dimensional manifoldM.

Choose an embeddingi:M →S^n+k normal bundleν(M).

Choose a tubular neighborhoodN ⊆S^n+k ofM. This is a compact manifold with boundary∂N withM⊆int(N)and comes with a diffeomorphismf: (Dν(M),Sν(M))−→^∼⁼ (N, ∂N)which is the identity on the zero section.

Letc:S^n+k →Th(ν(M))be the collaps map onto theThom space Th(ν(M)):=Dν(M)/Sν(M)which is given byf⁻¹on int(N)and sends any point outside int(N)to the base point.

Then the Hurewicz homomorphismπ_n+k(Th(M))→H_n+k(Th(M)) sends[c]to a generator of the infinite cyclic groupH_n+k(Th(M)).

(11)

Sν

Dν M

Collapse

Figure 1: Pontryagin-Thom construction

1

(12)

The normal bundle is stably independent of the choice of the embedding.

Next we describe the homotopy theoretic analog of the normal bundle for a finiten-dimensional Poincaré complexX.

Definition (Spivak normal structure)

ASpivak normal(k−1)-structureis a pair(p,c)wherep:E →X is a (k−1)-spherical fibration called theSpivak normal fibration, and c:S^n+k →Th(p)is a map such that the Hurewicz homomorphism h:π_n+k(Th(p))→H_n+k(Th(p))sends[c]to a generator of the infinite cyclic groupH_n+k(Th(p)).

(13)

Theorem (Existence and Uniqueness of Spivak Normal Fibrations)

1 If k is a natural number satisfying k ≥n+1, then there exists a Spivak normal(k−1)-structure(p,c);

2 For i =0,1let p_i:E_i →X and c_i:S^n+kⁱ →Th(p_i)be Spivak normal(k_i−1)-structures for X .

Then there exists an integer k with k ≥k₀,k₁such that there is up to strong fibre homotopy precisely one strong fibre homotopy equivalence

(id,f) :p₀∗S^k−k⁰ →p₁∗S^k−k¹ for whichπ_n+k(Th(f))(Σ^k−k⁰([c₀])) = Σ^k^−k¹([c₁])holds.

(14)

The Pontryagin-Thom construction yields aSpivak normal (k−1)-structureon a closed manifoldM with the sphere bundle Sν(M)as the spherical(k−1)fibration.

Hence a finiten-dimensional Poincaré complex is homotopy equivalent to a closed manifold only if the Spivak normal fibration has (stably) avector bundle reduction.

There exists a finiten-dimensional Poincaré complex whose Spivak normal fibration does not possess a vector bundle reduction and which therefore is not homotopy equivalent to a closed manifold.

Hence we assume from now on thatX is a (connected oriented) finiten-dimensional Poincaré complex which comes with a vector bundle reductionξ of the Spivak normal fibration.

(15)

Normal maps

Definition (Normal map of degree one)

Anormal map of degree onewith targetX consists of:

A closed (oriented)n-dimensional manifoldM;

A map of degree onef:M→X;

A(k+n)-dimensional vector bundleξoverX; A bundle mapf:TM⊕R^k →ξ coveringf.

(16)

A vector bundle reduction yields a normal map of degree one with X as target as explained next.

Letη be a vector bundle reduction of the Spivak normal fibration.

Letc:S^n+k →Th(p)be the associated collaps map. Make it transversal to the zero-section in Th(p).

LetM be the preimage of the zero-section. This is a closed submanifold ofS^n+k and comes with a mapf:M →X of degree one covered by a bundle mapν(M ⊆S^n+k)→η.

SinceTM⊕ν(M ⊆S^n+k)is stably trivial, we can construct from these data a normal map of degree one fromMtoX.

(17)

Problem (Surgery problem)

Let(f,f) :M →X be a normal map of degree one. Can we modify it without changing the target such that f becomes a homotopy

equivalence?

Exercise (Existence of normal maps)

Suppose that X is homotopy equivalent to a closed manifold M. Show that then there exists a normal map of degree one from M to X whose underlying map f:M→X is a homotopy equivalence.

(18)

The surgery step

Suppose thatM is a closed manifold of dimensionn,X is a CW-complex andf:M →X is ak-connected map. Consider ω∈π_k+1(f)represented by a diagram

S^k ^q ^//

j

M f

D^k+1 ^Q ^//X. We want to killω.

In the category ofCW-complexes this can be achieved by attaching cells. But attaching a cell destroys in general the structure of a closed manifold, so we have to do a more sophisticated modification.

(19)

Suppose that the mapq:S^k →Mextends to an embedding q^th:S^k×D^n−k ,→M.

Let int(im(q^th))be the interior of the image ofq^th. Then

M−int(im(q^th))is a manifold with boundary im(q^th|S^k×S^n−k−1).

We can get rid of the boundary by attachingD^k+1×S^n−k⁻¹along q^th|S^k×S^n−k−1. Denote the resulting manifold

M⁰ :=

D^k+1×S^n−k−1

∪q^th|_Sk_×Sn−k−1

M−int(im(q^th)) .

The manifoldM⁰is said to be obtained fromMbysurgery along q^th.

(20)

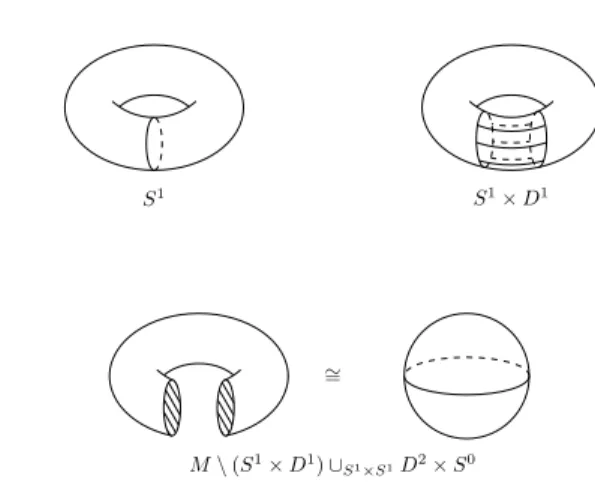
Letf:T²→S²be aHopf collapse map. We fixy₀∈S¹so that S¹:=S¹× {y₀} ⊂T²satisfiesf(S¹) =x₀. We defineω∈π₂(f)by extendingf|S¹ to the constant map atx₀on all ofD². The following diagram illustrates the effect of surgery on the source.

∼= M=T²

S¹ S¹×D¹

M\(S¹×D¹) M\(S¹×D¹)∪S¹×S¹D²×S⁰

Figure 1: Surgery step

(21)

Exercise (Surgery onT² →S²)

Show that the map f⁰:S²→S²obtained by carrying out the surgery step on the Hopf collapse map f:T²→S²as described above is a homotopy equivalence.

Exercise (Euler characteristic as surgery obstruction)

Consider a map f:M →X from a closed n-dimensional manifold M to a finite CW -complex X . Suppose that it can be converted by a finite sequence of surgery steps to a homotopy equivalence f⁰:M⁰ →X . Show that thenχ(M)−χ(X)≡0 mod 2.

(22)

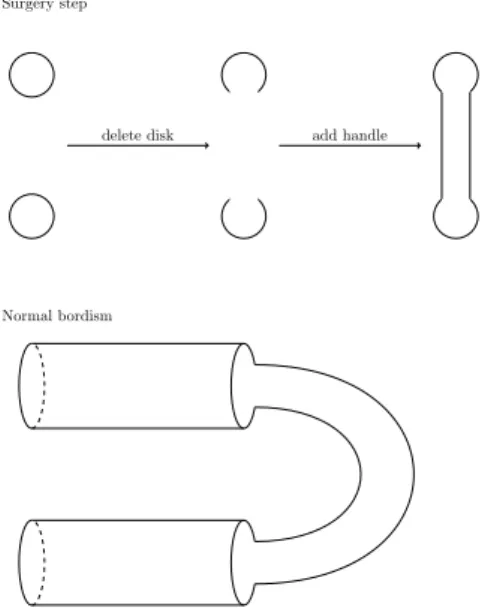
It is important to notice that the mapsf:M →X andf⁰:M⁰→X are bordant as manifolds with reference map toX.

The relevant bordism is given by W =

D^k+1×D^n−k

∪q^th(M×[0,1]),

where we think ofq^thas an embeddingS^k×D^n−k →M× {1}. In other words,W is obtained fromM×[0,1]by attaching a handle D^k+1×D^n−k toM× {1}.

ThenMappears inW asM× {0}andM⁰ as other component of the boundary ofW.

The manifoldW is called thetrace of surgeryalong the embeddingq^th.

(23)

delete disk add handle Surgery step

Normal bordism

Figure 1: normal bordism

1

(24)

Notice that the inclusionM−int(im(q^th))→M is

(n−k−1)-connected sinceS^k ×S^n−k−1→S^k ×D^n−k is

(n−k−1)-connected. Henceπ_l(f) =π_l(f⁰)forl≤k and there is an epimorphismπ_k+1(f)→π_k+1(f⁰)whose kernel containsω,

provided that 2(k+1)≤n.

The condition 2(k+1)≤ncan be viewed as a consequence of Poincaré duality. Roughly speaking, if we change something in a manifold in dimensionl, Poincaré duality also forces a change in dimension(n−l). This phenomenon is the reason why there are surgery obstructions to converting any mapf:M→X into a homotopy equivalence in a finite number of surgery steps for odd dimensionn.

The bundle data ensure that the thickeningq^thexists when we are doing surgery below the middle dimension. If one carries out the thickening in a specific way, the bundle data extend to the resulting normal map of degree one and we can continue the process.

(25)

Theorem (Making a normal map highly connected)

Given a normal map of degree one, we can carry out a finite sequence of surgery steps so that the resulting f⁰:N →X is k -connected, where n=2k or n=2k +1.

Exercise (Criterion for homotopy equivalence)

Show that a normal map of degree one which is k +1-connected, where n=2k or n=2k+1, is a homotopy equivalence.

(26)

Hence we have to make a normal map, which is already k-connected,k+1-connected in order to achieve a homotopy equivalence, wheren=2k orn=2k+1. Exactly here the surgery obstructionoccurs.

In odd dimensionn=2k+1 the surgery obstruction comes from the previous observation that by Poincare duality modifications in the(k+1)-th homology cause automatically (undesired) changes in thek-th homology.

In even dimensionn=2k one encounters the problem that the bundle data only guarantee that one can find an immersion with finitely many self-intersection points

q^th:S^k×D^k →M.

The surgery obstruction is the algebraic obstruction to get rid of the self-intersection points. Ifn≥5, its vanishing is indeed sufficient to convertq^th into an embedding.

(27)

One prominent necessary surgery obstruction is given in the case n=4k by the difference of thesignaturessign(X)−sign(M)since the signature is a bordism invariant and a homotopy invariant.

Ifπ1(M)is simply connected andn=4k fork ≥2, then the vanishing of sign(X)−sign(M)is indeed sufficient.

Ifπ1(M)is simply connected andnis odd andn≥5, there are no surgery obstructions.

(28)

Theorem (Existence problem in the simply connected case) Let X be a simply connected finite Poincaré complex of dimension n

1 Suppose that n is odd and n≥5. Then X is homotopy equivalent to a closed manifold if and only if the Spivak normal fibration has a reduction to a vector bundle.

2 Suppose n=4k ≥5. Then X is homotopy equivalent to a closed manifold if and only if the Spivak normal fibration has a reduction to a vector bundleξ:E →X such that

hL(ξ),[X]i=sign(X).

3 Suppose that n=4k +2≥5. Then X is homotopy equivalent to a closed manifold if and only if the Spivak normal fibration has a reduction to a vector bundle such that the Arf invariant of the associated surgery problem, which takes values inZ/2, vanishes.

(29)

Algebraic L-groups

In general there are surgery obstructions taking values in the so calledL-groupsLn(Z[π₁(M)]).

In even dimensionsL_n(R)is defined for a ring with involution in terms ofquadratic formsoverR, where thehyperbolic quadratic formsalways represent zero. In odd dimensionsL_n(R)is defined in terms of automorphisms of hyperbolic quadratic forms, or, equivalently, in terms of so calledformations.

TheL-groups are easier to compute thanK-groups since they are 4-periodic, i.e.,L_n(R)∼=L_n+4(R).

We have

L_n(Z)∼=







Z ifn=4k; Z/2 ifn=4k+2;

{0} ifn=2k+1.

(30)

The surgery obstruction is defined in all dimensions and is always a necessary condition to solve the surgery problem.

In dimensionn≥5 the vanishing of the surgery obstruction is sufficient.

In dimension 4 the methods of proof of sufficiency break down because the so calledWhitney trickis not available anymore which relies in higher dimensions on the fact that two embedded 2-disks can be made disjoint by transversality.

In dimension 3 problems occur concerning the effect of surgery on the fundamental group.

(31)

The surgery program

The surgery programaddresses the uniqueness problem whether two closed manifoldsMandN are diffeomorphic.

The idea is to construct anh-cobordism(W;M,N)with vanishing Whitehead torsion. ThenW is diffeomorphic to the trivial

h-cobordism overMand henceM andN are diffeomorphic.

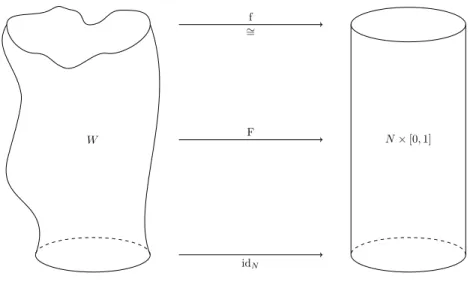
So thesurgery programis:

1 Construct a homotopy equivalencef:M →N;

2 Construct a cobordism(W;M,N)and a map

(F,f,id) : (W;M,N)→(N×[0,1];N× {0},N× {1});

3 ModifyW andF relative boundary by surgery such thatF becomes a homotopy equivalence and thusW becomes anh-cobordism.

4 During these processes one should make certain that the Whitehead torsion of the resultingh-cobordism is trivial. Or one knows already that Wh(π1(M))vanishes.

(32)

W

N M

N×[0,1]

f

∼=

F

idN

Figure 1: surgery program

(33)

The surgery exact sequence

Definition (The structure set)

LetNbe a closed topological manifold of dimensionn. We call two simple homotopy equivalencesf_i:M_i →N from closed topological manifoldsM_i of dimensionntoN fori =0,1 equivalent if there exists a homeomorphismg:M₀→M₁such thatf₁◦g is homotopic tof₀. Thestructure setSn^top(N)ofN is the set of equivalence classes of simple homotopy equivalencesM→X from closed topological manifolds of dimensionntoN. This set has a preferred base point, namely the class of the identity id:N →N.

If we assume Wh(π₁(N)) =0, then every homotopy equivalence with targetN is automatically simple.

There is an obvious version, where topological and

homeomorphism are replaced by smooth and diffeomorphism.

(34)

Definition (Topological rigid)

A closed topological manifoldN is calledtopologically rigidif any homotopy equivalencef:M →Nwith a closed manifoldM as source is homotopic to a homeomorphism.

Exercise (Topological rigidity)

Show for a closed topological manifold M that it is topologically rigid if and only if the structure setSn^top(M)consists of exactly one point.

Exercise (The sphere is topological rigidity)

Show that the Poincaré Conjecture implies that Sⁿis topologically rigid.

(35)

Theorem (The topological Surgery Exact Sequence)

For a closed n-dimensional topological manifold N with n≥5, there is an exact sequence of abelian groups, calledsurgery exact sequence,

· · ·−→ N^η _n+1^top(N×[0,1],N× {0,1})−→^σ L^s_n+1(Zπ)−→ S^∂ n^top(N)

−η

→ Nn^top(N)−→^σ L^s_n(Zπ)

L^s_n(Zπ)is the algebraicL-group of the group ringZπforpi =π1(N) (with decoration s).

Nn^top(N)is the set of normal bordism classes of normal maps of degree one with targetN.

N_n+1^top(N×[0,1],N× {0,1})is the set of normal bordism classes of normal maps(M, ∂M)→(N×[0,1],N× {0,1}of degree one with targetN×[0,1]which are simple homotopy equivalences on the boundary.

(36)

The mapσ is given by the surgery obstruction.

The mapηsendsf:M →Nto the normal map of degree one for whichξ = (f⁻¹)^∗TN.

The map∂sends an elementx ∈L_n+1(Zπ)tof:M→N if there exists a normal mapF: (W, ∂W)→(N×[0,1],N× {0,1}of degree one with targetN×[0,1]such that∂W =NqM, F|N =id_N,F|M =f, and the surgery obstruction ofF isx.

There is a spaceG/TOPtogether with bijections [N,G/TOP] −→ N^∼⁼ n^top(N);

[N×[0,1]/N× {0,1},G/TOP] −→ N^∼⁼ n+1^top(N×[0,1],N× {0,1}).

(37)

There is an analog of the surgery exact sequence in the smooth category except that it is only an exact sequence of pointed sets and not of abelian groups.

Corollary

A topological manifold of dimension n≥5is topologically rigid if and only if the mapNn+1^top(N×[0,1],N× {0,1})→L^s_n+1(Zπ)is surjective and the mapNn^top(N)→L^s_n(Zπ)is injective.

(38)

Notice the following formulas which look similar:

Ln(Z[Z]) ∼= Ln(Z)⊕Ln−1(Z);

Hⁿ(BZ) ∼= Hⁿ(pt)⊕ Hⁿ⁻¹(pt).

Question (L-theory of group rings and group homology) Is there a relation between Ln(RG)and group homology of G?