ÄHNLICHE DOKUMENTE
We demonstrate that scaling violations on the string tension can be considerably reduced by introducing effective coupling schemes, which allow for a safe extrapolation of AL, to
In a pure SU(N ) gauge theory in the fundamental representation without matter fields lattice simulations strongly indicate the presence of a finite temperature deconfinement
The muscles responsible for said changes where mainly the upper and lower leg muscles in the propulsion (higher contribution in the shod condition), the trunk muscles in the arm
The surrogate production process (SPP) conveys the same present discounted value of total output, uses the same present discounted value of labor input, and has the same average
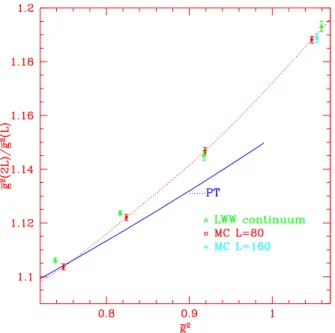
The running of the Schrödinger functional coupling from fourflavour lattice QCD with staggered quarks.. Paula Perez Rubio and
Thus, I employed the direct approach for an extensive study and obtained lattice results for the gluon momentum fraction on two different lattice ensembles, including an ensemble with
In this thesis we present results for the topological susceptibility χ YM , and investigate the property of factorization in the ’t Hooft large N limit of SU(N ) pure Yang-Mills
loop, as proposed in [6, 8], would have a higher ground state overlap than our current operators and therefore the effective potentials would reach the plateau-like region for